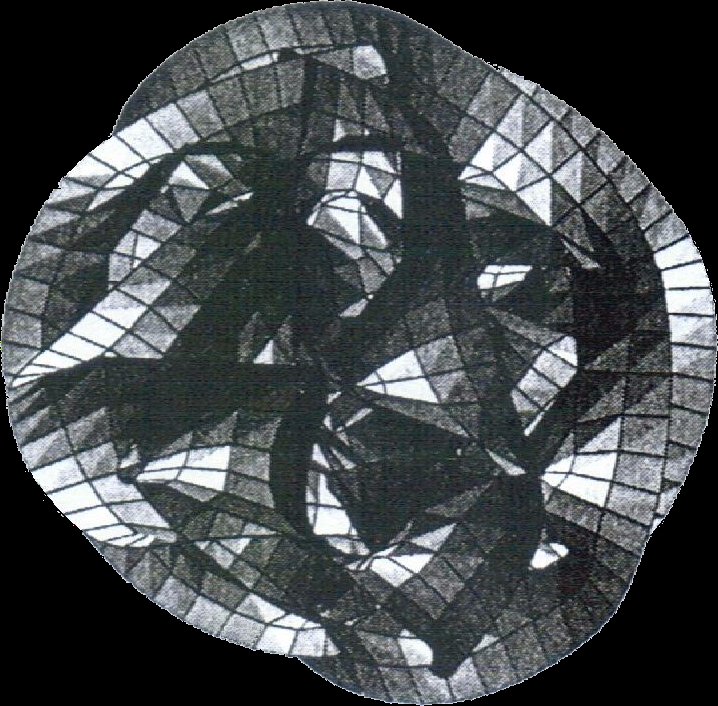
This is an example of a calabi-yau manifold, which is a 6-dimensional space that, according to
string theory, is a constituant of each point of our 3-dimensional space (in addition to our 3 "visible"
dimensions). Actually, 9 additional dimensions are required by string-theory for all the
math to resolve consistantly with the theory and observable reality. All 9 dimensions are accessed,
or passed through, by things (energy, particles) in our universe as they move/exist in our 3-space.
These dimensions are "tiny" and "curled" or "folded" up, as the illustration implies.
I wonder, though, if "size" or "scale" may actually be an illusion, which, if so, would make it
possible that our universe could be tiny and folded up, like a calabi-yau construct
in relation to another universe that is "inside" the calbi-yau dimensions, in which their primary dimensions
are as vast to their own perception as we percieve our own to be, and ours as condensed as we percieve theirs to be.
This would render the concept of "size" or "scale" as an irrelevant feature since both universes
are entwined: our universe is "tiny and folded up", thus mostly inaccessible to them, and theirs
is likewise "tiny and curled up", and mostly inaccessible, to ours. BUT, just how inaccessible ARE
these other dimensions, and how could we explore them without endangering their life-content? On a topological note: if our universe contains almost-infinite numbers of theirs, and their
universe contains almost-infinite numbers of ours, like a snake swallowing its own tail
how can we be inside of them and them inside of us? But I believe it is a possibility..."